Question

Two asteroids begin to gravitationally attract one another. If one asteroid has twice the mass of the other, which one experiences the greater force

Answer 1

F = G \frac{2 m^{2} }{r^{2} }

action and reaction forces with the same magnitude, but in the opposite direction, one applied to each body.

Step-by-step explanation:

The force between the asteroids is given by the law of universal gravitation

F = G m₁ m₂ / r²

in this case they give us the mass of each asteroid

m₁ = m

m₂ = 2m

we substitute

F = G m 2m / r²

F = G 2 m² / r²

`F = G (2 m^(2) )/(r^(2) )`

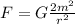
Let's analyze this expression, this is the force that one asteroid exerts on the other, therefore there are two forces, one applied to each asteroid, as the order of the products does not affect the result, the magnitude of the force is the same on each asteroid.

These are action and reaction forces with the same magnitude, but in the opposite direction, one applied to each body.

Consequently the two asteroid experiences the same magnitude of force