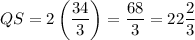Question

If r is the midpoint of qs, rs=2x-4, st=4x-1, and rt=8x-43, find qs

Answer 1

`\large\boxed{QS=22(2)/(3)}`

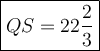
Explanation:

Look at the picture.

We must start from domain:

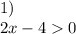`1)\\2x-4>0` add 4 to both sides

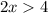`2x>4` divide both sides by 2

`x>2`

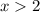
and

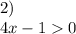`2)\\4x-1>0` add 1 to both sides

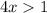`4x>1` divide both sides by 4

`x>(1)/(4)`

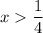
and

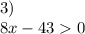`3)\\8x-43>0` add 43 to both sides

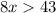`8x>43` divide both sides by 8

`x>(43)/(8)\\\\x>5(3)/(8)`

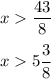
From 1, 2 and 3 we have:

`D:x>5(3)/(8)`

`\bold{(1)}\\\\(8x-43)+(4x-1)=2x-4\\8x-43+4x-1=2x-4\qquad\text{combine like terms}\\(8x+4x)+(-43-1)=2x-4\\12x-44=2x-4\qquad\text{add 44 to both sides}\\12x=2x+40\qquad\text{subtract}\ 2x\ \text{from both sides}\\10x=40\qquad\text{divide both sides by 10}\\x=4\\otin D`

`\bold{(2)}\\\\2x-4=(4x-1)-(8x-43)\\2x-4=4x-1-8x-(-43)\\2x-4=(4x-8x)+(-1+43)\qquad\text{combine like terms}\\2x-4=-4x+42\qquad\text{add 4 to both sides}\\2x=-4x+46\qquad\text{add}\ 4x\ \text{to both sides}\\6x=46\qquad\text{divide both sides by 6}\\x=(46)/(6)\\\\x=(23)/(3)\\\\x=7(2)/(3)\in D`

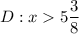
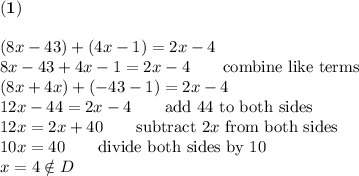
R is midpoint of QS. Therefore QR = RS ⇒ QS = 2(RS).

RS = 2x - 4

Put the value of x and calculate the length of RS:

`RS=2\left((23)/(3)\right)-4=(46)/(3)-(12)/(3)=(34)/(3)`

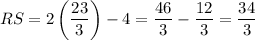
Therefore

`QS=2\left((34)/(3)\right)=(68)/(3)=22(2)/(3)`