Question

On a multiple-choice exam, there are 5 possible answers to each of 20 questions. Each question has only one correct answer. A student, “J”, has not studied and must guess the answer to each question (you may assume that the guesses are independent of each other). “J” must answer at least 10 questions correctly in order to pass the exam. The probability that “J” will pass the exam is

Answer 1

Explanation:

Given that on a multiple-choice exam, there are 5 possible answers to each of 20 questions.

Prob for correct guess if we do not know answer = one out of 5 = 0.20

Prob for wrong guess = 1-0.2 = 0.8

Each question is independent and there are only two outcomes.

X no of questions is Bin (20, 0.2)

Prob J passes = P(X≥10)

=P(X=10 or 11 or 12....20)

=
`\Sigma _10^20 20Cr (0.2)^r (0.8)^(20-r)`

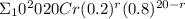
=0.00259

=0.0026