Answer:
The Cpk for the process is 1.61
Explanation:
The formula for Cpk is:
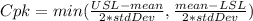
In this case in specific that we can test the capability of the process for 2 standard dev we modify the formula to adjust it:
so for this formula:
average: 23.546
USL: 23.23+0.612=23.842
LSL: 23.23-0.612=22.618
standard dev=0.092
So,
![Cpk=min((23.842-23.546)/(2*0.092) , (23.546-22.618)/(2*0.092) )\\[tex]Cpk=min(1.61 ,5.04 )\\\\Cpk=1.61](https://img.qammunity.org/2020/formulas/mathematics/college/dc9x8s65oacjr7goq1sdb3ur5kvdru4msg.png) \\\\[/tex]
\\\\[/tex]
The Cpk for the process is 1.61, so is a good indicator even with the control of two standard dev.