Question

Trey believes there is a correlation between the number of texts sent during class and GPA. He collected data and found that the line of best fit for his data can be modeled by the equation y = 3.8 − 0.4x.

Identify and interpret the slope in this scenario.

The slope is −0.4. Starting at 3.8, the GPA will decrease by 0.4 for every text sent in class.

The slope is 0.4. Starting at 3.8, the GPA will increase by 0.4 for every text sent in class.

The slope is −3.8. Starting at 0.4, the GPA will decrease by 3.8 for every text sent in class.

The slope is 3.8. Starting at 0.4, the GPA will increase by 3.8 for every text sent in class.

Answer 1

OPTION A (the first answer)

Explanation:

Answer 2

Answer: First Option

The slope is −0.4. Starting at 3.8, the GPA will decrease by 0.4 for every text sent in class.

Explanation:

The equation of a line has the following form

`y=mx+b`

Where m is the slope of the line and b is the intercept with the axis of the y

In this case, the equation is:

`y = 3.8 - 0.4x\\\\y=-0.4x+3.8`

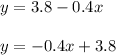
Observe that

`m = -0.4\\b = 3.8`

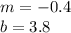
So if x represents the number of texts sent during classes, this means that
`x\geq 0`

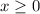
When x increases by one unit then y decreases -0.4. For example when
`x=1` then
`y=3.8-0.4=3.4`

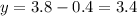
Then the greatest value it can take the GPA is 3.8. Therefore we can say that the function starts at y = 3.8.

The answer is the first option