Answer:
The critical t values are -1.746 and 1.746.
Explanation:
Given information:
The weight of a USB flash drive is 30 grams and is normally distributed.
Population mean = 30
Sample size = 17
Sample mean= 31.9
Standard deviation = 1.8
Significance level, α=0.10
Null hypothesis:

Alternative hypothesis:
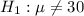
It is a two tailed test.
The t-critical values for a two-tailed test, for a significance level of α=0.1 are -1.746 and 1.746.
Therefore the critical t values are -1.746 and 1.746.