Step-by-step explanation:
It is given that,
Mass of the ball, m = 52 g = 0.052 kg
Initial speed of the ball, u = 28.5 m/s
Final speed of the ball, v = -15.5 m/s (it rebounds)
If the ball is in contact with the wall for 3.45 ms, t = 0.00345 s
Acceleration,
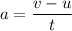
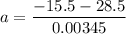

So, the average acceleration of the ball during this time interval is
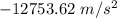 . Hence, this is the required solution.
. Hence, this is the required solution.