Answer:
Length of the arc of this sector, l = 14 cm
Step-by-step explanation:
It is given that, the perimeter of a sector of a circle is the sum of the two sides formed by the radii and the length of the included arc.
Perimeter of sector, P = 28 cm
Area of sector,
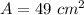
According to figure,
2r + l = 28 ............(1)
Area of sector,

Where,
 is in radian and
is in radian and
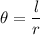
Since,
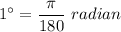
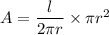
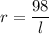
Put the value of r in equation (1) so,
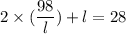
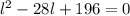
On solving above equation for l we get, l = 14 cm. So, the length of the arc of this sector is 14 cm. Hence, this is the required solution.