Answer:
The parametric equations of line are x=-8-t, y=5+8t and z=2+t.
Explanation:
It is given that the line passes through the point (−8, 5, 2) and is perpendicular to the plane given by −x + 8y + z = 5.
The coordinate of point are (−8, 5, 2).
Normal vector = <-1,8,1>
The position vector of a line is
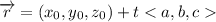
then the parametric equations of line are
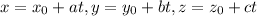 .
.
Where, (x_0,y_0,z_0) are the coordinate of point and <a,b,c> is normal vector.
The position vector of given line is
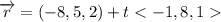
The parametric equations of line are

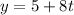

Therefore the parametric equations of line are x=-8-t, y=5+8t and z=2+t.