Question

Elwin Osbourne, CIO at GFS, Inc., is studying employee use of GFS e-mail for non-business communications. He plans to use a 98% confidence interval estimate of the proportion of e-mail messages that are non-business; he will accept a 0.05 error. Previous studies indicate that approximately 30% of employee e-mail is not business related. Elwin should sample _______ e-mail messages.

Answer 1

Answer: 456

Step-by-step explanation:

Given : Significance level :
`\alpha= 1-0.98=0.02`

Critical value :
`z_(\alpha/2)=2.33`

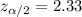
Margin of error :
`E=\pm0.05`

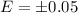
The prior proportion of employee e-mail is not business related:
`p=0.30`

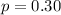
The formula to find the sample size is given by :-

`n=(p(1-p))((z_(\alpha/2))/(E))^2`

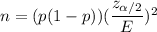
i.e.
`n=(0.3(0.7))(((2.33))/(0.05))^2=456.0276\approx456`

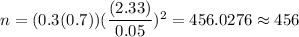
Hence, Elwin should sample 456 e-mail messages.