Question

Determine whether or not the vector field is conservative. If it is conservative, find a function f such that F = ∇f. (If the vector field is not conservative, enter DNE.) F(x, y, z) = xyz2i + x9yz2j + x9y2zk

Answer 1

We're looking for
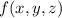`f(x,y,z)` such that
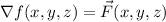`\\abla f(x,y,z)=\vec F(x,y,z)`, which requires

`(\partial f)/(\partial x)=xyz^2`

`(\partial f)/(\partial y)=x^9yz^2`

`(\partial f)/(\partial z)=x^9y^2z`

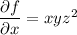
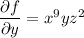
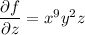
Integrating both sides of the first PDE wrt
`x` gives

`f(x,y,z)=\frac12x^2yz^2+g(y,z)`

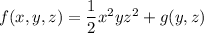
Differenting this wrt
`y` gives

`(\partial f)/(\partial y)=x^9yz^2=\frac12x^2z^2+(\partial g)/(\partial y)`

`\implies(\partial g)/(\partial y)=\frac12x^2z^2(2x^7y-1)`

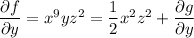
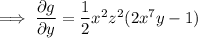
but we're assuming
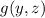`g(y,z)` is a function that doesn't depend on
`x`, which is contradicted by this result, and so there is no such
`f` and
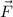`\vec F` is not conservative.