Answer:
The money invested in the account 1 was $25,000 and in the account 2 was 5,000.
Explanation:
In this case we can formulate a system of equations that could find the amount invested in each account, this is:
Money invested in the account 1 is A
Money invested in the account 2 is B
Eq. 1:
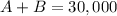
Eq. 2:
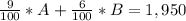
Replacing the equation 1 in 2, this is:
![(9)/(100) *(30,000-B)+(6)/(100) *B=1,950</p><p>Clearing the value of B:</p><p>[tex]2,700-0.09B+0.06B=1,950](https://img.qammunity.org/2020/formulas/mathematics/high-school/8qvfwk703xugkw8rq8zu4mu5o6t87sgy3n.png)
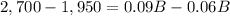
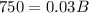
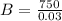
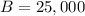
Now, we can find A:
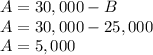
The money invested in the account 1 was $5,000 and in the account 2 was 25,000.