Answer:
pH of the solution is 11.24
Step-by-step explanation:
Total mass of caffeine in 225 mL solution upon dissolution of two tablets =
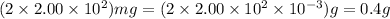
So molarity of caffeine in solution =
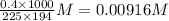
We have to construct an ice table to calculate change in concentration at equilibrium
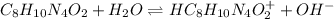
I:0.00916 0 0
C:-x +x +x
E:0.00916-x x x
So,
![([HC_(8)H_(10)N_(4)O_(2)^(+)][OH^(-)])/([C_(8)H_(10)N_(4)O_(2)])=K_(b)](https://img.qammunity.org/2020/formulas/chemistry/college/4ijv4u10qghyi16cs9rmcc5snz7r2p65o7.png)
Species inside third bracket represent equilibrium concentrations
So,
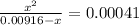
or,

Hence
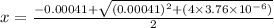
So,
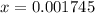 M
M
So,
![pH=14-pOH=14+log[OH^(-)]=14+logx=14+log(0.001745)=11.24](https://img.qammunity.org/2020/formulas/chemistry/college/rvnqt6917vrudyhr90e35h0haliq556pri.png)