Answer:
The minimum average cost per dulcimer is 1.8 hundred dollars,
1.5 hundred dulcimers should be built.
Step-by-step explanation:
Given function that represents the average cost ( in hundred of dollars ) per dulcimer,
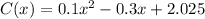
Differentiating with respect to x,
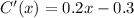
Again differentiating,
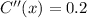
For maximum and minimum,
C'(x) = 0
0.2x - 0.3 = 0
0.2x = 0.3
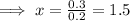
For x = 1.5,
C''(x) = positive,
Hence, C(x) is minimum for x = 1.5,
Minimum value = C(1.5) = 0.1(1.5)² - 0.3(1.5) + 2.025 = 0.1(2.25) - 0.45 + 2.025 = 1.8
Therefore, the minimum average cost per dulcimer is 1.8 hundred dollars for 1.5 hundred dulcimers.