Answer:
The value of BC is 30.
Explanation:
Given information: A, B, and C are collinear, B lies between A and C, AC = 48, AB = 2x+2, and BC = 3x+6.
If A, B, and C are collinear, B lies between A and C, then by using segment addition property, we get
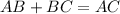
Substitute AC = 48, AB = 2x+2, and BC = 3x+6 in the above equation.
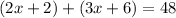
On combining like terms we get
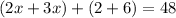
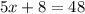
Subtract 8 from both sides.
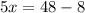
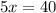
Divide 5 from both sides.
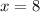
The value of x is 8.
We need to find the value of BC.
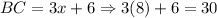
Therefore the value of BC is 30.