Answer:
Rotational kinetic energy of the forearm is 412.33 J.
Step-by-step explanation:
It is given that,
The linear velocity of the ball relative to the elbow joint is, v = 19.9 m/s
Distance from the joint, r = 0.49 m
Moment of inertia of the forearm, I = 0.5 kg/m²
We need to find the rotational kinetic energy of the forearm. It is given by :
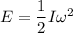
 is the angular speed,
is the angular speed,
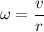

E = 412.33 J
So, the rotational kinetic energy of the forearm is 412.33 J. Hence this is the required solution.