Answer:
work done lifting the bucket (sand and rope) to the top of the building,
W=67.46 Nm
Step-by-step explanation:
in this question we have given
mass of bucket=20kg
mass of rope=

height of building= 15 meter
We have to find the work done lifting the bucket (sand and rope) to the building =work done in lifting the rope + work done in lifting the sand
work done in lifting the rope is given as,
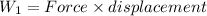
=
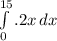 ..............(1)
..............(1)
=
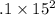
=22.5 Nm
work done in lifting the sand is given as,
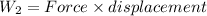
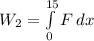 .................(2)
.................(2)
Here,
F=mx+c
here,
c=20-18
c=2
m=

m=.133
Therefore,
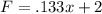
Put value of F in equation 2

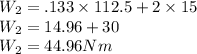
Therefore,
work done lifting the bucket (sand and rope) to the top of the building,
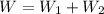
W=22.5 Nm+44.96 Nm
W=67.46 Nm