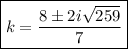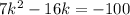
Add a squared constant to both sides:
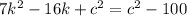
On the left, we wish to condense the quadratic into a squared binomial,
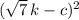
Expanding this gives
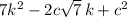
which tells us

Then
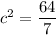 , and
, and
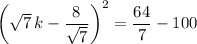
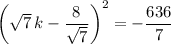
If you're looking for real-valued solutions, there are none, since the square root of a negative number doesn't exist... but if you're allowing complex-valued solutions, we can take the square root of both sides to get
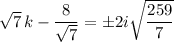
Multiply both sides by
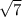 to eliminate denominators, then solve for
to eliminate denominators, then solve for
 :
: