Answer: 96.34%
Step-by-step explanation:
Half life is the amount of time taken by a radioactive material to decay to half of its original value.
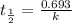
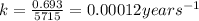
Expression for rate law for first order kinetics is given by:
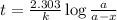
where,
k = rate constant
t = time for decomposition = 300 years
a = let initial amount of the reactant = 100
a - x = amount left after decay process = ?
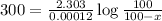

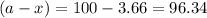
Thus 96.34 percent of a given amount remains after 300 years.