Answer:


Slant asymptote: y = x - 2
C) Removable discontinuity at x = -1 ⇒ (-1, ¹⁶/₃)
Infinite discontinuity at x = 2
Explanation:
Factor Theorem
If f(x) is a polynomial, and f(a) = 0, then (x – a) is a factor of f(x).
Therefore, if the polynomial f(x) has the given zeros of 7, -1 and -3 then:

Expanded:
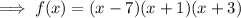
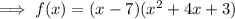
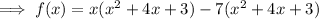


Part B
Factor
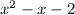 :
:
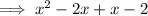
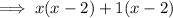
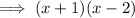
Therefore:
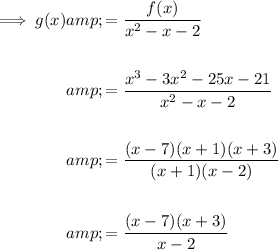
A slant asymptote occurs when the degree of the numerator polynomial is greater than the degree of the denominator polynomial.
To find the slant asymptote, divide the numerator by the denominator.
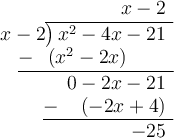
Therefore, the slant asymptote is y = x - 2.
Part C
Discontinuous function: A function that is not continuous.
Removable Discontinuity (holes): When a rational function has a factor with an x that is in both the numerator and the denominator.
Jump Discontinuity: The function jumps from one point to another along the curve of the function, often splitting the curve into two separate sections.
Infinite Discontinuity: When a function has a vertical asymptote (a line that the curve gets infinitely close to, but never touches).
Therefore, the discontinuities of function g(x) are:
- Removable discontinuity at x = -1 ⇒ (-1, ¹⁶/₃).
- Infinite discontinuity at x = 2.