Answer:
A, C
Explanation:
Actually, those questions require us to develop those equations to derive into trigonometrical equations so that we can unveil them or not. Doing it only two alternatives, the other ones will not result in Trigonometrical Identities.
Examining
A) True
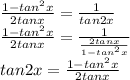
Double angle

B) False,
No further development towards a Trig Identity
C) True
Double Angle Sine Formula

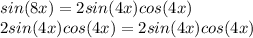
D) False No further development towards a Trig Identity
![[sin(x)-cos(x)]^(2) =1+sin(2x)\\ sin^(2) (x)-2sin(x)cos(x)+cos^(2)x=1+2sinxcosx\\ \\sin^(2) (x)+cos^(2)x=1+4sin(x)cos(x)](https://img.qammunity.org/2020/formulas/mathematics/high-school/jt2ijqvo1hf516mjobmbo62by5l6c7inyg.png)