Answer:
TRUE
Step-by-step explanation:
As we know that position of minimum intensity on the screen at a given angle of the screen is given by equation
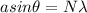
now for width of central maximum we will find the angular width of first minimum

now linear width of the central bright fringe is given as

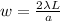
so we can say that if width of the central fringe will decrease by increasing the width of the slit as they as inversely dependent on each other
So its TRUE