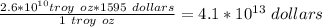Question

Earth's oceans have an average depth of 3800 m, a total surface area of 3.63 x 108 km2, and an average concentration of dissolved gold of 5.8 x 10?9 VI_ (a) How many grams of gold are in the oceans? {b} How many cubic meters of gold are in the oceans? (c). Assuming the price of gold is $1595/troy oz, what is the value of gold in the oceans (1 tray oz = 311 g: dof gold =19.3 g/cm3)?

Answer 1

a) Mass of gold in ocean = 8.1*10¹² g

b) Volume of gold = 4.2*10¹¹ cm³

c) Value of gold = $ 4.1*10¹³

Step-by-step explanation:

a) Depth of ocean = 3800 m

Surface area of ocean = 3.63*10⁸ km²

Now, 10⁶ m² = 1 km²

Therefore, the area in units of m² = 3.63*10¹⁴ m²

`Volume\ of\ ocean = Depth*Area = 3800m*3.63*10^(14) m2=1.4*10^(18) m3`

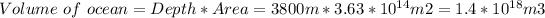
Average concentration of gold in ocean = 5.8*10⁻⁹ g/L

Now, 1 g/L = 1000 g/m³

Therefore, concentration of gold in g/m³ = 5.8*10⁻⁶ g/m³

`Mass\ of\ gold =1.4*10^(18) m^(3) *5.8*10^(-6) g/m3 = 8.1*10^(12) g`

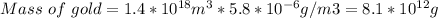
b) Mass of gold present = 8.1*10¹² g

Density of gold = 19.3 g/cm³

`Volume\ of\ gold = (Mass)/(density) =(8.1*10^(12) )/(19.3) =4.2*10^(11) cm^(3)`

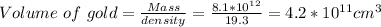
c) Price of gold = $1595/troy oz

Unit conversion

1 troy oz = 311 g

Therefore, 8.1*10¹² g of gold is equivalent to:

`(8.1*10^(12) g* 1\  troy\ oz)/(311g) =2.6*10^(10) \ troy\ oz`

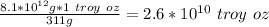
The value of gold in the ocean is:

`(2.6*10^(10) troy\ oz*1595\ dollars)/(1\ troy\  oz) =4.1*10^(13) \ dollars`