Question

Last year Janet purchased a $1,000 face value corporate bond with an 10% annual coupon rate and a 30-year maturity. At the time of the purchase, it had an expected yield to maturity of 12.69%. If Janet sold the bond today for $1,004.57, what rate of return would she have earned for the past year? Do not round intermediate calculations. Round your answer to two decimal places.

Answer 1

RoR = 38.74%

Step-by-step explanation:

We need to know the value of the bond at the moment of the purchase.

That will be the present value of the coupon payment and the maturity date. Both measurement should be done using 12.69 rate

`C * (1-(1+r)^(-time) )/(rate) = PV\\`

C 50 (1,000 x 10% /2) The bond pay semiannually

time 60 (30 years and 2 payment per year)

rate 0.06345 (12.69 annual rate / 2 = 6.345% semiannually rate)

`50 * (1-(1+0.06345)^(-60) )/(0.06345) = PV\\`

PV $768.3652

`(Maturity)/((1 + rate)^(time) ) = PV`

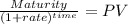
Maturity 1000

time 30

rate 0.1269

`(1000)/((1 + 0.1269)^(30) ) = PV`

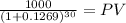
PV $27.7612

PV c $768.3652

PV m $27.7612

Total $796.1264

Proceeds: 1,004.57 sales price + 100 cuopon payment for the year

It purchase at 796.1264

`(proceeds)/(investment) -1 = $Rate of Return`

`(1,104.57)/(796.1264) -1 = 0.387430362`

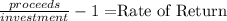
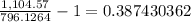
RoR = 38.74%