Question

Cost, revenue, and profit are in dollars and x is the number of units. Suppose that the marginal revenue for a product is MR = 1500 and the marginal cost is MC = 30 x + 4 , with a fixed cost of $900. (a) Find the profit or loss from the production and sale of 5 units.

Answer 1

Profit 6,130

Step-by-step explanation:

MC = 30X + 4

when X=5

Cost to produce 5 units:

We will need to calcualte the MC for 1, 2 , 3, 4 and 5 units and then add them together

MC = 30(5) + 4 = 150 + 4 = 154

MC = 30(4) + 4 = 150 + 4 = 124

MC = 30(3) + 4 = 150 + 4 = 94

MC = 30(2) + 4 = 150 + 4 = 64

MC = 30(1) + 4 = 150 + 4 = 34

Total 470

Giving this, now anther way, more easy would be to use the Gauss method to a summatory:

`S=(n*(n+1))/(2)`

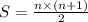
S to 5 from 1 of (30x+4) =

`30 * (5*6)/(2) +4 * 5`

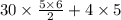
S = 470

Now we can continue:

Total Marginal cost 470 + Fixed Cost: 900 = 1370

MR = 1500 revenue for adding 1 unit

1500 x 5 = 7500 total revenue

total revenue - total cost = profit

7500 - 1370 = 6,130