Question

The waiting times between a subway departure schedule and the arrival of a passenger are uniformly distributed between 0 and 8 minutes. Find the probability that a randomly selected passenger has a waiting time greater than 3.25 minutes. Find the probability that a randomly selected passenger has a waiting time greater than 3.25 minutes.

Answer 1

Answer: 0.5625

Explanation:

Given : The waiting times between a subway departure schedule and the arrival of a passenger are uniformly distributed between 0 and 8 minutes.

The density function for uniform distribution :-

`f(x)=(1)/(8-0)=(1)/(8)`

The required interval : (3.25,8)=8-3.5=4.5

The probability that a randomly selected passenger has a waiting time greater than 3.25 minutes is given by :-

`=(4.5)/(3.25)=0.5625`

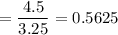
Hence, the probability that a randomly selected passenger has a waiting time greater than 3.25 minutes