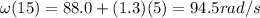Question

A wheel with radius 32 cm is rotating at a rate of 14 rev/sA wheel with radius 32 cm is rotating at a rate of 14 rev/s(a) What is the angular speed in radians per second?(b) In a time interval of 5 s, what is the angle in radians through which the wheel rotates?(c) At t=10 s the angular speed begins to increase at a rate of 1.3 rad/s/s. At t=15 s, what is the angular speed in radians per second?

Answer 1

(a) 88.0 rad/s

The angular speed of the wheel is

`\omega = 14 rev/s`

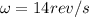
Keeping in mind that

1 revolution =
`2\pi` radians

The angular speed in radians/second can be found by solving the proportion:

`14 rev/s : x = 1 rev : 2 \pi rad`

From which we find

`x=(14\cdot 2 \pi)/(1)=88.0 rad/s`

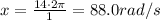
(b) 440 radians

Assuming the wheel is rotating at constant angular speed, the angular displacement of the wheel at time t is given by

`\theta= \omega t`

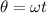
where

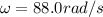`\omega=88.0 rad/s` is the angular speed

t is the time

Substituting

t = 5 s

we find the angle through which the wheel has rotated after 5 seconds:

`\theta=(88.0)(5)=440 rad`

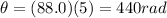
(c) 94.5 rad/s

The angular speed after a time t is given by

`\omega(t) = \omega_o + \alpha t`

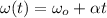
where

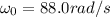`\omega_0=88.0 rad/s` is the angular speed at t=10 s, when the acceleration starts

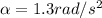`\alpha = 1.3 rad/s^2` is the angular acceleration

The duration of the acceleration is

t = 15 s - 10 s = 5 s

So substituting this value into the equation, we find the new angular speed:

`\omega(15) = 88.0+(1.3)(5)=94.5 rad/s`