Answer:
Part 67)
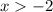 and
and
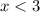
Part 68)
 or
or
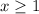
Explanation:
Part 67) we know that
The solution of the number line is the interval -------> (-2,3)
All real numbers greater than -2 and less than 3
so
The compound inequality could be
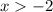 and
and
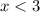
"And” indicates that, both statements must be true at the same time
Part 68) we know that
The solution of the number line is the interval (-∞,-1] ∪ [1,∞)
All real numbers less than or equal to -1 or all real numbers greater than or equal to 1
The compound inequality could be
 or
or
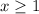
"Or” indicates that, as long as either statement is true, the entire compound sentence is true