Question

Cynthia had a credit card with a 17% APR and a $3,265 balance. She had budgeted to have the credit card paid off in 24 months. But after missing a single monthly payment, Cynthia’s credit card company has increased her interest rate to 21%. How much extra will Cynthia have to pay in finance charges (interest) because of the increase in her APR if she still pays off the credit card in 24 months?

Answer 1

a

Explanation:

Answer 2

Cynthia will have to pay $152.16 extra in finance charges.

Explanation:

Cynthia had a credit card with a 17% APR and a $3,265 balance.

Cynthia’s credit card company has increased her interest rate to 21%.

Here we have two scenarios, 1st when p = 3265 r = 17% and n = 24

Second when p = p = 3265 r = 21% and n = 24

Scenario 1:

`r =17/12/100=0.014166`

EMI formula is :

`(p* r*(1+r)^(n))/((1+r)^(n)-1)`

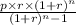
Putting the values in formula we get;

`(3265*0.014166*(1+0.014166)^(24))/((1+0.014166)^(24)-1)`

=
`(3265*0.014166*(1.014166)^(24))/((1.014166)^(24)-1)`

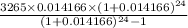
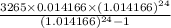
EMI = $161.43

Scenario 2:

r =
`21/12/100=0.0175`

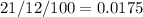
Putting the values in formula we get;

`(3265*0.0175*(1+0.0175)^(24))/((1+0.0175)^(24)-1)`

=
`(3265*0.0175*(1.0175)^(24))/((1.0175)^(24)-1)`

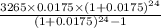
EMI = $167.77

Now, difference in EMI's =
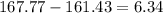`167.77-161.43=6.34` dollars

And for 24 months this amount becomes =
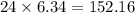`24*6.34=152.16` dollars

Therefore, Cynthia will have to pay $152.16 extra in finance charges.