Answer:
The largest area that can be enclosed is 9248 square feet.
Explanation:
This is a typical problem of optimization that can be solved using derivatives. We have a rectangular region, and let us denote the height by
 , and the base by
, and the base by
 .
.
Then, the area of the rectangle is
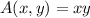 . Notice that the area is a function of
. Notice that the area is a function of
 and
and
 , but if we want to use calculus, we should have only one variable. This can be done if we find a relationship between both variables.
, but if we want to use calculus, we should have only one variable. This can be done if we find a relationship between both variables.
Recall that the fences will not bu used in the whole perimeter of the rectangular area, but only in three sides. Hence,
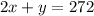 . (Without lost of generality we can consider
. (Without lost of generality we can consider
 , instead.)
, instead.)
Then,
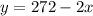 ans substituting in the formula for the area:
ans substituting in the formula for the area:
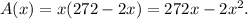
Taking derivative with respect to x:
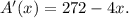
Its only zero can be found solving the equation
 . Hence, its only zero of
. Hence, its only zero of
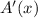 is
is
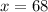 . In order to assure that 68 is a point of maximum, we find
. In order to assure that 68 is a point of maximum, we find
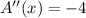 and conclude that, in effect, 68 is a point of maximum.
and conclude that, in effect, 68 is a point of maximum.
We obtain the value of
 substituting the value of
substituting the value of
 in the relationship between both variables:
in the relationship between both variables:
 . With the values of
. With the values of
 and
and
 we can calculate the desired area:
we can calculate the desired area:
