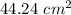For this case we have that the area of the shaded region is given by the subtraction of areas, area of the circle minus the area of the rectangle. That is to say:
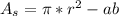
Where:
A: It is the radius of the circle
a, b: They are the sides of the rectangle
According to the data we have:

Taking
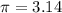
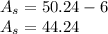
So, the area of the shaded region is
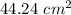
Answer: