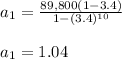Question

Find a1 if Sn = 89,800, r = 3.4, and n = 10. Round to the nearest hundredth if necessary.

Answer 1

a1=1.04

Explanation:

Answer 2

`a_1=1.04`

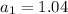
Explanation:

We have a geometric sequence with:

`Sn = 89,800`,
`r = 3.4`, and
`n = 10`

Where

Sn is the sum of the sequence

r is the common ratio

`a_1` is the first term in the sequence

n is the number of terms in the sequence

The formula to calculate the sum of a finite geometric sequence is:

`S_n=(a_1(1-r^n))/(1-r)`

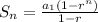
Then:

`89,800=(a_1(1-(3.4)^(10)))/(1-3.4)`

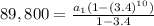
Now we solve for
`a_1`

`89,800(1-3.4)=a_1(1-(3.4)^(10))`

`a_1=(89,800(1-3.4))/(1-(3.4)^(10))\\\\a_1=1.04`