Question

Find the EQUATION of the line with exact numbers

Answer 1

You need to know where the line crosses the y axis (vertical) - AKA the y intercept, and the slope of the line.
The y intercept is 5. The slope is -1/3 because every time the line goes down one square, it also goes right three squares.
Equation:
y=mx+b
where m is the slope and b is the y intercept
y = 1/3x + 5
Another way to write the same thing
y = x/3 + 5
Both correct!

Answer 2

`\large\boxed{y=-1/3x+5}`

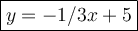
Explanation:

In this question, we're trying to find the equation of the given line.

In this case, we would represent the line as:

y = mx + b

m = slope

b = beginning point

Lets find the equation.

We know that the beginning point is on the y-axis on the graph.

We can see that 5 is on the y-axis. Therefore, our beginning point would be 5.

Your equation should look like this:

y = mx + 5

Now, lets find the slope.

We know that the line is decreasing, so it would be a negative slope.

To find the slope, we would use the rise over run strategy. In this case, we would go down and run since it's a negative.

We go down 1 and go to the right 3 times. This means that our slope of the line would be represented as a fraction.

The slope of the line would be 1/3

When you put it into the equation, it should look like:

y = -1/3x + 5

The equation of the line is y = -1/3x + 5

I hope this helped you out.

Good luck on your academics.

Have a fantastic day!