Question

Technetium-99m has a half-life of 6.01 hours. If a patient injected with technetium-99m is safe to leave the hospital once 75% of the dose has decayed, when is the patient allowed to leave?

Answer 1

The patient is allowed to leave after 12.02 hours from the time of ingestion of the medicine.

Step-by-step explanation:

`\lambda =\frac{0.693}{t_{(1)/(2)}}`

`A=A_o* e^(-\lambda t)`

Where:

`\lambda`= decay constant

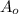`A_o` =concentration left after time t

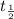`t_{(1)/(2)}` = Half life of the sample

Half-life of Technetium-99m =
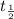`t_{(1)/(2)}`= 6.01 hours.

`\lambda =(0.693)/(6.01 hour)=0.1153 (hour)^(-1)`

`A_o=x`

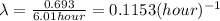
75% of medic en must have been decayed before leaving the hospital. Then percentage of left over concentration of medicine will be: 100% - 75% = 25%

`A=25\%of x=0.25x`

Time elapsed during this process= t

`0.25x=x* e^{-0.1153 (hour)^(-1)* t}`

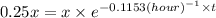
t = 12.02 hours

The patient is allowed to leave after 12.02 hours from the time of ingestion of the medicine.