Given:
Vertices of a triangle are D(0,5), E(2,0) and F(-4,2).
To find:
The intersection point of medians DG and EH.
Solution:
We know that, intersection point of all the medians of a triangle is called centroid.
The formula of centroid is

Vertices of a triangle are D(0,5), E(2,0) and F(-4,2). So, the centroid of the triangle is

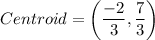
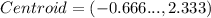
Round each coordinate to the nearest tenth.
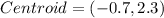
Therefore, the correct option is C.