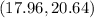Question

Assuming the population of interest is approximately normally​ distributed, construct a 95​% confidence interval estimate for the population mean given the values below. x overbar=19.3 s=3.1 n=23 The 95​% confidence interval for the population mean is from nothing to nothing.

Answer 1

Answer:
`(17.96,20.64)`

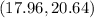
Explanation:

The confidence interval for population mean is given by :-

`\overline{x}\ \pm\ t_(n-1,\alpha/2)(s)/(√(n))`

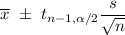
Given :
`\overline{x}=19.3`

`s= 3.1`

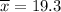
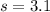
n=23, which is a small sample(n<30), so we use t-test.

Significance level:
`1-0.95=0.05`

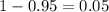
Critical value :
`t_(n-1,\alpha/2)=t_(22,0.025)=2.074`

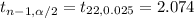
Then , the confidence interval for population mean will be :-

`19.3\ \pm\ (2.074)(3.1)/(√(23))\\\\\approx19.3\pm1.34\\\\=(19.3-1.34,19.3+1.34)\\\\=(17.96,20.64)`

Hence, the 95​% confidence interval for the population mean is
`(17.96,20.64)`