Question

Darcie wants to crochet a minimum of 3 blankets to donate to a homeless shelter. Darcie crochets at a rate of 1/15 of a blanket per day. She has 60 days until when she wants to donate the blankets, but she also wants to skip crocheting some days so she can volunteer in other ways.

Write an inequality to determine the number of days, s, Darcie can skip crocheting and still meet her goal.

Please help. I'm on a time limit. Plus this is worth 30 points

Answer 1

The inequality is:

`s\leq 60-(3 / (1)/(15))`

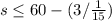
which can be simplified into:

`s\leq 15`

Step-by-step explanation:

Assume the following:

s is the number of days Darcie can skip crocheting

t is the total number of days left before donation = 60 days

r is the number of days required to crochet 3 blankets

Now, for the given problem:

We are given that Darcie crochets at a rate of 1/15 of a blanket per day

We start by getting the number of days required to crochet 3 blankets

Number of day required =
`3` ÷
`(1)/(15)`
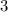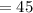`= 45` days

The number of days Darcie can skip must be less than or equal to the difference between the total number of days left and the number of days required to crochet the 3 blankets

s ≤ t - r

`s \leq 60 - (3 / (1)/(15))\\  \\ s\leq 60 - 45\\ \\ s\leq 15`

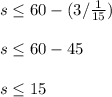
The above inequality means that Darcie can crocheting at most 15 days in order to be able to meet her target

Hope this helps :)