Answer:
B. 283.45 cubic inches.
Explanation:
We know that a volleyball has spherical form.
To find the volume of a sphere, we have the following formula
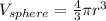
Now, we know that the radius is defined as half the diameter
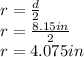
Then, we replace the radius to find the volume of the ball
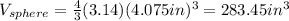
Therefore, the right answer is B. 283.45 cubic inches.