Question

A hiker in Africa discovers a skull that contains 32% of its original amount of C-14. find the age of the skull.

Answer 1

9419.3 years.

Explanation:

Let the initial amount of C-14 be 100 units.

We have been given that a hiker in Africa discovers a skull that contains 32% of its original amount of C-14. We are asked to find the age of the skull.

We will use half life formula to solve our given problem.

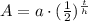`A=a\cdot((1)/(2))^{(t)/(h)}`, where,

A = Amount left after t years,

a = Initial amount,

t = time,

h = Half life.

We know that half-life of C-14 is 5730 years.

32% of 100 units would be 32.

`32=100\cdot((1)/(2))^{(t)/(5730)}`

`(32)/(100)=\frac{100\cdot((1)/(2))^{(t)/(5730)}}{100}`

`0.32=(0.5)^{(t)/(5730)}`

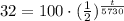
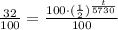
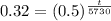
Now, we will take natural log of both sides.

`\text{ln}(0.32)=\text{ln}((0.5)^{(t)/(5730)})`

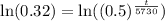
Using log property
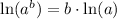`\text{ln}(a^b)=b\cdot\text{ln}(a)`, we will get:

`\text{ln}(0.32)=(t)/(5730)\cdot \text{ln}(0.5)`

`\frac{\text{ln}(0.32)}{\cdot \text{ln}(0.5)}=\frac{t\cdot \text{ln}(0.5)}{5730\cdot \text{ln}(0.5)}`

`1.64385618977=(t)/(5730)`

`(t)/(5730)=1.64385618977`

`(t)/(5730)*5730=1.64385618977*5730`

`t=9419.295967`

`t\approx 9419.3`

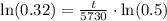
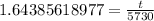
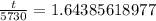
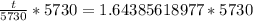
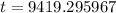
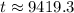
Therefore, the age of skull is approximately 9419.3 years.