Answer: Option C
Discontinuity at (−4, −2), zero at (−2, 0)
Explanation:
We have the following expression:

Note that the function is not defined for x = -4, since the division by zero is not defined
We factor the expression of the numerator.
We look for two numbers that when you multiply them you obtain as a result 8, and by adding both numbers you get as a result 6.
You can check that the numbers that meet these requirements are 4 and 2.
So the factors of the quadratic function are:

So
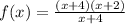 with
with
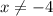
By simplifying the expression we have:
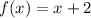 with
with
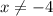
Since the function is not defined for x = -4 then f(x) has a discontinuity at the point (-4, -2)
To find the zero of the function you must equal f (x) to zero and solve for x
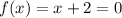
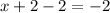

The zero of the function is: (-2, 0)
The answer is the Option C