Answer:
10.791 kJ
Step-by-step explanation:
Given that,
Mass of the skier, m = 58 kg
Initial position, h₁ = 24 m
Final position, h₂ = 19 m
We need to find the kinetic energy do they have at the top of the second hill.
Using the conservation of energy for both positions.
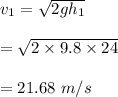
And
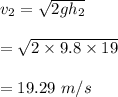
Kinetic energy :
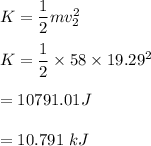
So, the kinetic energy they have at the top of the second hill is 10.791 kJ.