Question

An FAS calibration curve (absorbance, y-axis vs. FAS in g/mL) gave an equation he regression line of: y-3678(x)+0.056. If an unknown FAS sample solution gave a reading of 25.6 %, what is the FAS concentration in the measured solution?

Answer 1

FAS concentration = 1.61*10^-4M

Step-by-step explanation:

Beer Lambert's law relates the absorbance (A) of a substance to its concentration (c) as:

`A = \epsilon lc----(1)`

where ε = molar absorption coefficient

l = path length

A plot of 'A' vs 'c' gives a straight line with slope = εl

In addition absorbance (A) is related to % Transmittance (%T) as:

A = 2-log%T----(2)

For the FAS solution, the corresponding calibration fit is given as:

y = 3678(x) + 0.056

This implies that the slope = εl = 3678

It is given that %T = 25.6%

`A = 2-log(25.6)=0.592`

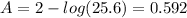
Based on equation(1):

`c = (A)/(\epsilon l)=(0.592)/(3678)=1.61*10^(-4)M`