Question

Solve the system by substitution.

y=x^2+4x+1
y=x+1

A. (1,2),(5,6)

B. (-1.0),(3/2,5,2)

C. (0,1),(-3,-2)

D. (3,4),(1,2)

Answer 1

C

Explanation:

We can substitue equation 2 into equation because y is common in both equations:

1. y=x^2+4x+1

2. y=x+1

therefore:

`x+1=x^2+4\cdot{x}+1`

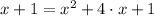
We can take x+1 to the right side:

`0=x^2+3\cdot{x}`

We can write it as multiples of x:

`0=x\cdot{(x+3)}`

Therefore the answer is x=0 or x=-3 and therefore we can substitute into equation 1 and 2. therefore for equation 1 and 2 when x=0

`y=1`

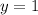
and when x=-3

`y=-2`

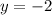
The answer is (0,1) and (-3,-2) which is C

Answer 2

C.(0,1),(-3,-2)

Explanation:

(x+1)=x^2+4x+1

0=x^2+3x

(x+3)(x+0)

x=-3 x=0

when x=-3, y=(3)^2+4(-3)+1=-2

when x=0, y=0^2+0x+1=1