Answer: 0.105
Explanation:
Binomial probability formula :-
 , here P(X) is the probability of getting success in x trials , n is total trials and p is the probability of getting success in each trial.
, here P(X) is the probability of getting success in x trials , n is total trials and p is the probability of getting success in each trial.
Given : The random variable x denotes the number of votes who favor a certain candidate for mayor.
Sample size : n=18
The probability that city's voters favor the candidate: p=0.43
Now, the probability that exactly 10 of the sampled voters favor the candidate is given by :-
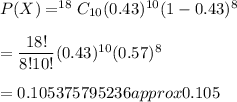
Hence, the probability that exactly 10 of the sampled voters favor the candidate = 0.105