Question

a motorcycle traveling on the highway at a velocity of 120 kilometre-per-hour passes a car traveling at a velocity of 90 kilometre-per-hour from the point of view of a passenger on the car what is the velocity of a motorcycle​

Answer 1

The relative velocity of the motorcycle to a passenger in the car is 30 km/h

Step-by-step explanation:

The question relates to the principle of relative velocity and reference frames

The given parameters are;

The velocity of the motorcycle, v₁ = 120 km/h

The velocity of the car, v₂ = 90 km/h

The relative velocity of an object X with regards to another object Y is the velocity the object X will seem to be moving with to an observer in the rest frame of object Y written as
`\underset{v}{\rightarrow}_(X|Y)` =
`\underset{v}{\rightarrow}_(X)` -
`\underset{v}{\rightarrow}_(Y)`

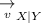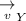
Therefore, the relative velocity of the motorcycle to the car is
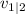`v_(1|2)` = v₁ - v₂, which give;

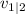`v_(1|2)` = 120 km/h - 90 km/h = 30 km/h

The relative velocity of the motorcycle to a passenger in the car = 30 km/h.