If we can't go past the hundreths digits, then out numbers look like 0.71, 0.72, 0.73...
So, our number lies between 0.75 and 0.76. We have to see which one is closer, i.e. we have to subtract the numbers:
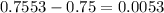
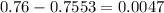
Since 0.0047 is smaller than 0.0053, we deduce that 0.76 is closer to 0.7553, and thus it's a better approximation.