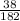Answer:
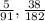
Explanation:
(a)Probability that these three marbles are all red
choosing 3 red out of 6 is given by
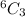
and choosing 3 marble from 14 marbles=

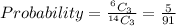
(b)All of same color
It is possible when they are of either red or green
For red it is
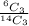
For Green it is
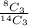
total=

=