Question

A ball is bouncing to a height that is 1/3 of the previous height after every bounce. What kind of sequence is this? How could you find the total distance travelled by the ball?

Answer 1

Answer and Explanation:

The ball is bouncing to a height of 1/3 of its previous height this is a type of geometric sequence the total distance can be found by the sum of geometric sequence

For example let the initial height is 243 fit

After one bounce it will reach 243/3 =81 feet

After second bounce 81/3=27 feet

After third bounce 27/3 =9 feet

After fourth bounce 9/3 =3 feet

So a sequence is formed that is 243,81,27,9,3..........

Here
`r=(3)/(9)=(1)/(3)`

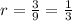
Sum of infinite GP =
`(a)/(1-r)`

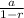
From this formula we can find the total distance traveled by the ball