Question

An AC source has an output rms voltage of 86.0 V at a frequency of 75.0 Hz. The source is connected across a 28.5-mH inductor. (a) Find the inductive reactance of the circuit. (b) Find the rms current in the circuit. (c) Find the maximum current in the circuit.

Answer 1

A) The reactance of the inductor is given by:

X = 2πfL

X = reactance, f = source frequency, L = inductance

Given values:

f = 75.0Hz, L = 28.5×10⁻³H

Plug in and solve for X:

X = 2π(75.0)(28.5×10⁻³)

X = 13.4Ω

B) V = IZ

V = rms source voltage, I = rms current, Z = impedance

Given values:

V = 86.0V

With just the inductor in the circuit, Z = X = 13.4Ω

Plug in and solve for I:

86.0 = I(13.4)

I = 6.42A

C) The rms current is given by:

`I_(rms) = I_(max)/√(2)`

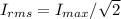
We have
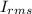`I_(rms)` = 6.42A so plug that in and solve for
`I_(max)`:

`6.42 = I_(max)/√(2)`

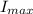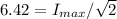
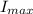`I_(max)` = 9.08A