Question

Three friends went to the fair. Michelle ate three hot dogs, one pretzel, and two snow cones. She spent $25. Amanda ate two hot dogs, three pretzels, and three snow cones. She spent $30. Lucas ate two hot dogs, two pretzels, and four snow cones. He spent $25. Which system of equations matches the friends' night at the fair?

2x + y + 3z = 25
2x + 3y + 3z = 30
2x + 2y + 4z = 25
3x + y + 2z = 25
2x + 3y + 3z = 30
2x + 2y + 4z = 25
3x + y + 2z = 25
3x + 2y + 3z = 30
2x + 2y + 4z = 25
3x + y + 2z = 25
2x + 3y + 3z = 30
2x + 4y + 2z = 25

Answer 1

the answer to your question is 2x + 3y + 3z = 30

Answer 2

b.
`3x+y+2z=25[/tex</strong>]</p><p><strong>[tex]2x+3y+3z=$30`

`2x+2y+4z=$25`

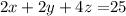
Explanation:

We are given that three friends went to fair

Let cost of hot dog=x

Cost of pretzel=y

Cost of snow cone=z

Michelle ate 3 hot dog,one pretzel and two snow cones and spent $25.

Therefore, according to question the equation is

Lucas ate two hot dogs,two pretzel and four snow cones and spent $25.

Therefore, the equation is

`2x+2y+4z=$25`

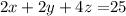
Hence, option b is true.